1. Craps Dice Roll Odds
2. Craps Dice Rolling Strategy
3. Craps Dice Roll Videos Live

Craps dice are 6-sided. When you roll 2 of these dice, you have 11 possible totals and 36 possible outcomes: A total of 2, which is a combination of 1-1. The main components of a good dice roll include developing ultimate control over the dice and understanding the various craps dice sets and their purposes. Typically, dice rolls are random, and players cannot involve into games of craps without losing cash because they play by the rules and house edge established by the casino.

This is the 2^nd in a series of posts explaining the game of craps in detail. The 1^st post was about the staff working at the craps table. This post is about how the table layout works and what it means for the player.

Part 2 of 6

• 1 The Craps Dealers and Other Staff at the Dice Table: Who’s Who
• 3 How to Play a Craps Game in a Casino
• 4 The Best and Worst Craps Bets You Can Make
• 5 Craps Bets Ranked According to House Edge
• 6 How (and Why) to Act Like a Craps Player

Craps in a casino is played at a big table with a green felt layout. This is where you place your bets.

But not all the bets are labeled on the layout.

• The All Sevens Set is the most popular dice set currently being used at the craps tables on the Come-Out roll. It has the ‘seven’ showing on all sides and if executed correctly, it will give you the best chance of hitting a natural (7) or (11) and winning on the Come-Out.
• Craps is a game where many bettors get to stake their chips on a roll of the dice. The table layout makes the game seem a little confusing at first, but the rules are easy to pick up with experience. Before stepping up to a table, read about the kinds of bets available and how a round of craps play out.
• Craps is a dice game in which the players make wagers on the outcome of the roll, or a series of rolls, of a pair of dice. Players may wager money against each other (playing 'street craps') or a bank (playing 'casino craps', also known as 'table craps', or often just 'craps').

And some of the bets that aren’t labeled are important. You’ll learn why soon.

A Closer Look at the Craps Table

When I say the craps table is big, I mean it’s bigger than most people expect until they’ve seen one in person. It’s about 5 feet wide. And it’s often as long as a Toyota Camry.

But the size can vary from casino to casino, too.

Wooden sides surround the surface of the craps table, but 2 spots, in particular, are left open—one for the stickman and one for the boxman.

Unlike roulette, there are no seats at a craps table. It’s played standing. The only person sitting at a craps table is the boxman. Depending on the size of the table, it can host between a dozen and 20 players at a time.

The insides of the wooden sides are coated with foam rubber in some places and mirrors in others. The foam rubber ensures a random roll of the dice, while the mirrors make it easier for the boxman and the floorman to view the action and the results of the rolls.

The dice are always somewhere on the table in plain sight. This prevents cheating on the part of the casino and the players.

The Layout of the Craps Table Is Even More Important

That felt tabletop has bets printed on it. It’s usually green, although you’ll also often see tables covered in red felt. Some casinos try to use their branding on the table, and they might change the color in use based on that, too.

Craps Dice Roll Odds

The lines which divide the tabletop into areas are almost always white, regardless of the color of the rest of the table. The bets are printed in various colors—red, white, and/or yellow.

If you look at a picture of a craps table, you’ll notice 3 sections:

• A section on one end
• A section on the other end
• A middle section

The 2 sections on the end are identical. The casino sets the table up this way so you can make bets with the standing dealers from either end of the table.

The outermost labeled bet on the table is the pass-line bet. It’s usually just labeled “PASS LINE.” The section for this bet runs from the edge of the table to the center of the table. This is the most commonly placed bet at the craps table.

Parallel and next to the pass-line bet is the don’t pass bet. Most people like to root for the shooter, so they don’t place the don’t pass bet, even though it has slightly better odds than the pass-line bet.

Next to where it says “don’t pass,” the table usually has printed “bar 12” or “bar 2.” This means that if the roll is a 12 (or a 2), the don’t pass bet doesn’t pay—it’s treated as a push. You get to keep your original bet, but you don’t get any winnings.

A future post will explain what these bets are in detail, but for now, I want you to understand where they’re located on the craps table.

You’ll also see an area labeled “COME.” This is where you place a “come bet,” which is another of the most popular and useful bets on the table. Many players ignore this bet because they don’t understand what it is or how it works. When you finish reading this series of posts, you won’t have that problem.

Another bet is available—the opposite of the come bet. It’s called the “don’t come bet.” It’s also printed with the words “bar 2” or “bar 12” next to it.

The other big area on the craps table is labeled “FIELD.” This is where you can place field bets. Besides the word “FIELD,” you’ll also see several numbers in that section: 2, 3, 4, 9, 10, 11, or 12. The 2 and the 12 are in circles, too. (You win that bet if you placed a bet on any of those numbers and one of them come up on the next roll. The circled numbers usually pay off double or triple.)

Lots of beginners like the field bet, but it’s a sucker bet. Skip it. I’ll explain why soon.

A couple more bets are also big and bold on the surface: “BIG 6” and “BIG 8.” These are sucker bets, too. Skip them. If you want to bet on 6 or 8, there are better ways – offering better odds.

The place bets also have a big section devoted to them. The numbers listed in that section, each of which is in its own box, are 4, 5, 6, 8, 9, and 10. 6 and 9 are usually spelled out using text (“six” and “nine,” to prevent confusion—an upside down 6 looks like a 9 and vice versa.)

This is where the buck is placed after a point is set by the shooter.

Those are the basic wagers found at either end of the table. I’ll go into more detail about what those bets mean, what they pay off, and how big the house edge is for each of them in the appropriate post.

The best bet on the table isn’t labeled at all, though. It’s called the “free odds bet” or just the “odds bet.” I’ll explain it soon, too.

The Bets in the Center of the Table

You’ll find a dizzying array of bets in the center of the table, and these are the bets that the stickman manages. You can place hard way bets, any craps, 7, or 11, among others.

I’ll explain all these bets in detail, too, but for now, I want to give you this advice:

Ever.

The house edge is the number we use to measure how advantageous a bet is for the casino. The higher the house edge, the bigger the casino’s mathematical advantage is over you.

The bets in the center of the table all have a house edge of at least 9%. Some of them are much higher than that.

Many of the bets on the 2 ends of the table are lousy, too.

But smart craps players stick with the best bets on the table. You can get the house edge below 1% if you place the right bets.

You Also Need to Know about the Dice

Craps Dice Rolling Strategy

Obviously, you need some understanding of the table layout, but you also need an understanding of how the dice work and the various combinations they can create.

Casino dice are almost perfect cubes. And when I say “almost perfect,” we’re talking about dice that are made with unimaginable precision. The cubes are ¾ of an inch wide. The corners of these dice are sharp because of the precise angles involved. You don’t want to step on them barefoot. You also don’t want someone throwing them at you.

These dice are larger than dice used at home in games like Yahtzee. They’re generally red and translucent so you can see that there are no weights or anything inside them. They’re also imprinted with the casino’s logo.

It’s important to the casino that the dice are kept honest.

I mentioned this in my previous post, but if a shooter’s on a winning streak, the boxman will examine the dice to make sure they haven’t been switched out or tampered with.

Dice Combinations

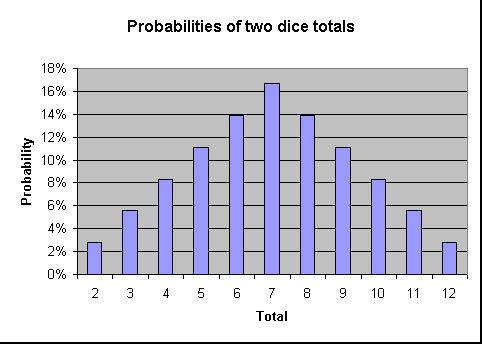
Craps dice are 6-sided. When you roll 2 of these dice, you have 11 possible totals and 36 possible outcomes:

• A total of 2, which is a combination of 1-1. (There’s only one way to roll a 2.)
• A total of 3, which is a combination of 1-2 or 2-1. (There are 2 ways to roll a 3.)
• A total of 4, which is a combination of 1-3, 2-2, or 3-1. (There are 3 ways to roll a 4.)
• A total of 5, which is a combination of 1-4, 2-3, 3-2, or 4-1. (There are 4 ways to roll a 5.)
• A total of 6, which is a combination of 1-5, 2-4, 3-3, 4-2, or 5-1. (There are 5 ways to roll a 6.)
• A total of 7, which is a combination of 1-6, 2-5, 3-4, 4-3, 5-2, or 6-1. (There are 6 ways to roll a 7.)
• A total of 8, which is a combination of 2-6, 3-5, 4-4, 5-3, or 6-2. (There are 5 ways to roll an 8.)
• A total of 9, which is a combination of 3-6, 4-5, 5-4, or 6-3. (There are 4 ways to roll a 9.)
• A total of 10, which is a combination of 4-6, 5-5, or 6-4. (There are 3 ways to roll a 10.)
• A total of 11, which is a combination of 5-6 or 6-5. (There are 2 ways to roll an 11.)
• A total of 12, which is a combination of 6-6. (There is only one way to roll a 12.)

You’ll notice if you look carefully that this is a classic bell curve. The 7 is in the middle of the curve, and it’s the most likely result. The 6 and the 8 are the 2^nd most likely results, placing them to either side of the 7 on the curve, and so on.

Point Numbers

Some of these totals are “point numbers.” They’re the 4, 5, 6, 8, 9, and 10.

The 7 is the important reference point for the point numbers, because the odds of rolling a point number are measure against the odds of rolling a 7.

There are 3 ways to roll a 4 or a 10.

Craps Dice Roll Videos Live

There are 6 ways to roll a 7.

The probability of a 7 coming up before a 4 or a 10 is 6/3, or 2 to 1.

There are 4 ways to roll a 5 or a 9.

There are 6 ways to roll a 7.

The probability of a 7 coming up before a 5 or a 9 is 6/4, or 3 to 2.

There are 5 ways to roll a 6 or an 8.

There are 6 ways to roll a 7.

The probability of a 7 coming up before a 6 or an 8 is 6/5, or 6 to 5.

Those are really important odds to understand, as will become clear soon.

Other Odds for Other Totals and Outcomes

The way the casino makes its money is by paying off your bets at odds that aren’t commensurate with the odds of winning.

The odds of rolling a total of 11 are 17 to 1. (There are 17 ways to fail for every 1 way to succeed.)

But if you bet that the total on the next roll will be 11 and you win, you only get paid at 15 to 1.

Suppose you placed that bet 18 times, at $100 each. You’d win $1500 on the one time you succeeded, but on the other 17 rolls, you’d lose $1700. You’d have a net loss of $200 over 18 spins.

That’s an average of $11.11 lost per spin, or 11.11%.

And that’s the house edge on that bet—11.11%.

You can always calculate these odds by remembering that probability is just a fraction that compares how many ways something can happen with how many ways it can’t happen.

For example, the odds of rolling a 2 (or a 12) are 35 to 1. There are 35 ways to lose, and only one way to win.

The odds of rolling a 3 (or an 11) are 17 to 1. There are 34 ways to lose and only 2 ways to win.

The odds of rolling a 4 (or a 10) are 11 to 1. There are 33 ways to lose and only 3 ways to win.

Conclusion

This is the 2^nd post in our series about playing craps and winning. The next post explains in detail exactly how the game of craps is played. This includes stuff like who rolls the dice, what happens after they roll the dice, and so on.

But I wanted to give you a solid background on both the casino employees involved in the game and the equipment used during the game. I also wanted to give you an introduction to how the math works when rolling a pair of dice.dd

Please enable JavaScript to view the comments powered by Disqus.

Alter then so that one die has a six on every side, and the other one has all ones and fives.

Thanks for the kind words. No, I don't think that wishful thinking helps in the casino, all other things being equal.
The question on the dice influence is a hotly debated topic. Personally, I'm very skeptical. As I review this reply in 2013 I still have yet to see convincing evidence anybody can influence enough to have an advantage.

I'm very skeptical of it. I go over some of the experiments on the topic in my craps appendix 3.

I don’t believe in it. So far I have yet to see a name I respect endorse the method, nor any evidence that it works. While I don’t entirely rule out the possibility I am extremely skeptical of it. I may live in Nevada but when it comes to things like dice setting I’m from Missouri, 'show me' it works.

With ordinary dice, the like those you get in a board game, this is true. However casino dice have inlaid spots. At the factory they drill holes for the spots then insert white colored spots into the holes, of the same density as the die itself. So the die is essentially a perfect cube. Even if they did use ordinary dice from a board game I doubt the bias would be nearly enough to overcome the house edge.

I think there is no such thing as a naturally bad shooter. With the possible exception of a few pros all dice throws can be considered truly random. There are seminars on how to overcome the house edge in craps by precession throwing but I make no claims for or against them. I have yet to see enough evidence either way.

I lost the $1800 to another gambling writer, not Stanford. I would have preferred more rolls but there was an obvious time contraint. Assuming one throw per minute it would take 34.7 days to throw the dice 50,000 times. I wasn’t the one who decided on 500 but it seemed like a reasonable compromise between a large sample size and time. You are right that 500 is too few to make a good case for or against influencing the dice, but 500 throws is better than zero.

For large numbers of throws we can use the Gaussian Curve approximation. The expected number of sevens in 655 throws is 655 × (1/6) = 109.1667. The variance is 655 × (1/6) × (5/6) = 90.9722. The standard deviation is sqr(90.9722) = 9.5379. Your 78 sevens is 109.1667 − 78 = 31.1667 less than expectation. This is (31.1667 - 0.5)/9.5379 = 3.22 standard deviations below expectation. The probability of falling 3.22 or more standard deviations south of expectations is 0.000641, or 1 in 1,560. I got this figure in Excel, using the formula, normsdist(-3.22).

This is about controlling the dice at Craps. You previously discussed the Stanford Wong Experiment, stating, 'The terms of the bet were whether precision shooters could roll fewer than 79.5 sevens in 500 rolls of the dice. The expected number in a random game would be 83.33. The probability of rolling 79 or fewer sevens in 500 random rolls is 32.66%.... The probability of rolling 74 or fewer sevens in 500 random rolls is 14.41%.'

The question I have about this bet is that 14.41% still isn’t 'statistically significant' [ i.e. p < 0.05 ] , which is usually taken to mean greater than two Standard Deviations from the Mean -- or a probability of less than a *combined* 5% of the event happening randomly on EITHER end of the series.

How many Sevens would have to be rolled in 500 rolls before you could say that there is a less than 2.5% chance that the outcome was entirely random (i.e. that the outcome was statistically significant) ?

Many Thanks & BTW , yours is ABSOLUTELY the BEST web site on the subject of gambling odds & probabilities that I’ve found .... keep up the good work !!!

Thank you for the kind words. You should not state the probability that the throws were non-random is p. The way it should be phrased is the probability that a random game would produce such a result is p. Nobody expected 500 rolls to prove or disprove anything. It wasn’t I who set the line at 79.5 sevens, but I doubt it was chosen to be statistically significant; but rather, I suspect the it was a point at which both parties would agree to the bet.

The 2.5% level of significance is 1.96 standard deviations from expectations. This can be found with the formula =normsinv(0.025) in Excel. The standard deviation of 500 rolls is sqr(500*(1/6)*(5/6)) = 8.333. So 1.96 standard deviations is 1.96 * 8.333 = 16.333 rolls south of expectations. The expected number of sevens in 500 throws is 500*(1/6) = 83.333. So 1.96 standard deviations south of that is 83.333 − 16.333 = 67. Checking this using the binomial distribution, the exact probability of 67 or fewer sevens is 2.627%.

There is no definitive point at which confidence is earned. It is a matter of degree. First, I would ask what is being tested for, and what the shooter estimates will happen. With any test there are two possible errors. A skilled shooter might fail, because of bad luck, or a random shooter might pass because of good luck. Of the two, I would prefer to avoid a false positive. I think a reasonable test would set the probability of a false negative at about 5%, and a false positive at about 1%.

For example, suppose the claimant says he can average one total of seven every seven throws of the dice. A random shooter would throw one seven every six throws, on average. By trial and error I find that a test meeting both these criteria would be to throw the dice 3,600 times, and require 547 or fewer sevens to pass, or one seven per 6.58 rolls.

A one in seven shooter should average 514.3 sevens, with a standard deviation of 21.00. Using the Gaussian approximation, the probability of such a skilled shooter throwing 548 or more sevens (a false negative) is 5.7%. A random shooter should average 600 sevens, with a standard deviation of 22.36. The probability of a random shooter passing the test (a false positive) is 0.94%. The graph below shows the possibe results for skilled and random shooters. If the results are to the left of the green line, then I would consider the shooter to have passed the test, and I would bet on him.

The practical dilemma is if we assume two throws per minute, it would take 30 hours to conduct the test. Perhaps I could be more liberal about the significance level, to cut down the time requirement, but the results would not be as convincing. I do think the time has come for a bigger test than the 500-roll Wong experiment.

First of all, she rolled the dice a total of 154 times, with the 154th roll being a seven out (Source: NJ.com). However, that does not mean she never rolled a seven in the first 153 rolls. She could have rolled lots of them on come out rolls. As I show in my May 3, 2003 column, the probability of making it to the 154th roll is 1 in 5.6 billion. The odds of winning Mega Millions are 1 in combin(56,5)*46 = 175,711,536. So going 154 rolls or more is about 32 times as hard. Given enough time and tables, which I think exist, something like this was bound to happen sooner or later. So, I wouldn't suspect cheating. I roughly estimate the probability that this happens any given year to be about 1%.

Also see my solution, expressed in matrices, at mathproblems.info, problem 204.

I think some of the casinos in Las Vegas are using dice that are weighted on one side. As evidence, I submit the results of 244 throws I collected at a Strip casino. What are the odds results this skewed could come from fair dice?

Dice Test Data
Dice Total	Observations
2	6
3	12
4	14
5	18
6	23
7	50
8	36
9	37
10	27
11	14
12	7
Total	244

7.7%.

The chi-squared test is perfectly suited to this kind of question. To use the test, take (a-e)²/e for each category, where a is the actual outcome, and e is the expected outcome. For example, the expected number of rolls totaling 2 in 244 throws is 244×(1/36) = 6.777778. If you don’t understand why the probability of rolling a 2 is 1/36, then please read my page on dice probability basics. For the chi-squared value for a total of 2, a=6 and e=6.777778, so (a-e)²/e = (6-6.777778)²/6.777778 = 0.089253802.

Chi-Squared Results

Dice Total	Observations	Expected	Chi-Squared
2	6	6.777778	0.089253
3	12	13.555556	0.178506
4	14	20.333333	1.972678
5	18	27.111111	3.061931
6	23	33.888889	3.498725
7	50	40.666667	2.142077
8	36	33.888889	0.131512
9	37	27.111111	3.607013
10	27	20.333333	2.185792
11	14	13.555556	0.014572
12	7	6.777778	0.007286
Total	244	244	16.889344

Then take the sum of the chi-squared column. In this example, the sum is 16.889344. That is called the chi-squared statistic. The number of 'degrees of freedom' is one less than the number of categories in the data, in this case 11-1=10. Finally, either look up a chi-squared statistic of 10.52 and 10 degrees of freedom in a statistics table, or use the formula =chidist(16.889344,10) in Excel. Either will give you a result of 7.7%. That means that the probability fair dice would produce results this skewed or more is 7.7%. The bottom line is while these results are more skewed than would be expected, they are not skewed enough to raise any eyebrows. If you continue this test, I would suggest collecting the individual outcome of each die, rather than the sum. It should also be noted that the chi-squared test is not appropriate if the expected number of outcomes of a category is low. A minimum expectation of 5 is a figure commonly bandied about.

Whether or not it is called a valid roll depends on where you are. New Jersey gaming regulation 19:47-1.9(a) states:

A roll of the dice shall be invalid whenever either or both of the dice go off the table or whenever one die comes to rest on top of the other. -- NJ 19:47-1.9(a)

Pennsylvania has the exact same regulation, Section 537.9(a):

A roll of the dice shall be invalid whenever either or both of the dice go off the table or whenever one die comes to rest on top of the other. -- PA 537.9(a)

I asked a Las Vegas dice dealer who said that here it would be called a valid roll, if it was otherwise a proper throw. Although he has never seen it happen, he said if it did the dealers would simply move the top die to see what number the lower die landed on. However, one can determine the outcome of the lower die without touching, or looking through, the top die. Here is how to do it. First, by looking at the four sides you can narrow down the possibilities on top to two. Here is how to tell according to the three possibilities.

• 1 or 6: Look for the 3. If the high dot is bordering the 5, the 1 is on top. Otherwise, if it is bordering the the 2, the 6 is on top.
• 2 or 5: Look for the 3. If the high dot is bordering the 6, the 2 is on top. Otherwise, if it is bordering the the 1, the 5 is on top.
• 3 or 4: Look for the 2. If the high dot is bordering the 6, the 3 is on top. Otherwise, if it is bordering the the 1, the 4 is on top.

This question was raised and discussed in the forum of my companion site Wizard of Vegas.

This question was asked at TwoPlusTwo.com, and was answered correctly by BruceZ. The following solution is the same method as that of BruceZ, who deserves proper credit. It is a difficult answer, so pay attention.

1. First, consider the expected number of rolls to obtain a total of two. The probability of a two is 1/36, so it would take 36 rolls on average to get the first 2.

2. Next, consider the expected number of rolls to get both a two and three. We already know it will take 36 rolls, on average, to get the two. If the three is obtained while waiting for the two, then no additional rolls will be needed for the 3. However, if not, the dice will have to be rolled more to get the three.

   The probability of a three is 1/18, so it would take on average 18 additional rolls to get the three, if the two came first. Given that there is 1 way to roll the two, and 2 ways to roll the three, the chances of the two being rolled first are 1/(1+2) = 1/3.

   So, there is a 1/3 chance we'll need the extra 18 rolls to get the three. Thus, the expected number of rolls to get both a two and three are 36+(1/3)×18 = 42.

3. Next, consider how many more rolls you will need for a four as well. By the time you roll the two and three, if you didn't get a four yet, then you will have to roll the dice 12 more times, on average, to get one. This is because the probability of a four is 1/12.

   What is the probability of getting the four before achieving the two and three? First, let's review a common rule of probability for when A and B are not mutually exclusive:

   pr(A or B) = pr(A) + pr(B) - pr(A and B)

   You subtract pr(A and B) because that contingency is double counted in pr(A) + pr(B). So,

   pr(4 before 2 or 3) = pr(4 before 2) + pr(4 before 3) - pr(4 before 2 and 3) = (3/4)+(3/5)-(3/6) = 0.85.

   The probability of not getting the four along the way to the two and three is 1.0 - 0.85 = 0.15. So, there is a 15% chance of needing the extra 12 rolls. Thus, the expected number of rolls to get a two, three, and four is 42 + 0.15*12 = 43.8.

4. Next, consider how many more rolls you will need for a five as well. By the time you roll the two to four, if you didn't get a five yet, then you will have to roll the dice 9 more times, on average, to get one, because the probability of a five is 4/36 = 1/9.

   What is the probability of getting the five before achieving the two, three, or four? The general rule is:

   pr (A or B or C) = pr(A) + pr(B) + pr(C) - pr(A and B) - pr(A and C) - pr(B and C) + pr(A and B and C)

   So, pr(5 before 2 or 3 or 4) = pr(5 before 2)+pr(5 before 3)+pr(5 before 4)-pr(5 before 2 and 3)-pr(5 before 2 and 4)-pr(5 before 3 and 4)+pr(5 before 2, 3, and 4) = (4/5)+(4/6)+(4/7)-(4/7)-(4/8)-(4/9)+(4/10) = 83/90. The probability of not getting the four along the way to the two to four is 1 - 83/90 = 7/90. So, there is a 7.78% chance of needing the extra 7.2 rolls. Thus, the expected number of rolls to get a two, three, four, and five is 43.8 + (7/90)*9 = 44.5.

5. Continue with the same logic, for totals of six to twelve. The number of calculations required for finding the probability of getting the next number before it is needed as the last number roughly doubles each time. By the time you get to the twelve, you will have to do 1,023 calculations.

   Here is the general rule for pr(A or B or C or ... or Z)

   pr(A or B or C or ... or Z) =
   pr(A) + pr(B) + ... + pr(Z)
   - pr (A and B) - pr(A and C) - ... - pr(Y and Z) Subtract the probability of every combination of two events
   + pr (A and B and C) + pr(A and B and D) + ... + pr(X and Y and Z) Add the probability of every combination of three events
   - pr (A and B and C and D) - pr(A and B and C and E) - ... - pr(W and X and Y and Z) Subtract the probability of every combination of four events

   Then keep repeating, remembering to add probability for odd number events and to subtract probabilities for an even number of events. This obviously gets tedious for large numbers of possible events, practically necessitating a spreadsheet or computer program.

The following table shows the the expected number for each step along the way. For example, 36 to get a two, 42 to get a two and three. The lower right cell shows the expected number of rolls to get all 11 totals is 61.217385.

Expected Number of Rolls Problem

Highest Number Needed	Probability	Expected Rolls if Needed	Probability not Needed	Probability Needed	Expected Total Rolls
2	0.027778	36.0	0.000000	1.000000	36.000000
3	0.055556	18.0	0.666667	0.333333	42.000000
4	0.083333	12.0	0.850000	0.150000	43.800000
5	0.111111	9.0	0.922222	0.077778	44.500000
6	0.138889	7.2	0.956044	0.043956	44.816484
7	0.166667	6.0	0.973646	0.026354	44.974607
8	0.138889	7.2	0.962994	0.037006	45.241049
9	0.111111	9.0	0.944827	0.055173	45.737607
10	0.083333	12.0	0.911570	0.088430	46.798765
11	0.055556	18.0	0.843824	0.156176	49.609939
12	0.027778	36.0	0.677571	0.322429	61.217385

This question was raised and discussed in the forum of my companion site Wizard of Vegas.

The Wizard says that website sounds like a lot of ranting and raving with no credible evidence whatsoever to justify the accusation. I'd be happy to expose any casino for using biased dice, if I had any evidence of it.
If anybody has legitimate evidence of biased dice, I'd be happy to examine it and publish my conclusions. Evidence I would like to see are either log files of rolls or, better yet, some actual alleged biased dice.
Furthermore, if the casinos really were using dice that produced more than the expected number of sevens, then why aren't these detectives privy to the conspiracy out there betting the don't pass and laying the odds?

The Hot Roll bonus round on slot machines awards the player the following number of coins according to the total of two dice. The player keeps collecting until he rolls a total of seven, which ends the bonus. If he rolls a seven on the first roll, he gets a consolation prize of 70 coins. Following are the prizes for all other totals besides seven:

• 2 or 12: 1,000
• 3 or 11: 600
• 4 or 10: 400
• 5 or 9: 300
• 6 or 8: 200

My question is what is average bonus win?

Click the following button for the answer.

Click the following button for the solution.

Let x be the answer. As long as the player doesn't roll a seven he can always expect future wins to be x, in addition to all previous wins. In other words, there is a memory-less property to throwing dice in that no matter how many rolls you have already thrown you are no closer to a seven than you were when you started.
I won't go into the basics of dice probabilities but just say the probability of each total is as follows:

• 2: 1/36
• 3: 2/36
• 4: 3/36
• 5: 4/36
• 6: 5/36
• 7: 6/36
• 8: 5/36
• 9: 4/36
• 10: 3/36
• 11: 2/36
• 12: 1/36

Before considering the consolation prize, the value of x can be expressed as:

x = (1/36)*(1000 + x) + (2/36)*(600 + x) + (3/36)*(400 + x) + (4/36)*(300 + x) + (5/36)*(200 + x) + (5/36)*(200 + x) + (4/36)*(300 + x) + (3/36)*(400 + x) + (2/36)*(600 + x) + (1/36)*(1000 + x)

Next, multiply both sides by 36:

36x = (1000 + x) + 2*(600 + x) + 3*(400 + x) + 4*(300 + x) + 5*(200 + x) + 5*(200 + x) + 4*(300 + x) + 3*(400 + x) + 2*(600 + x) + (1000 + x)
36x = 11,200 + 30x
6x = 11,200
x = 11,200/6 = 1866.67.
Next, the value of the consolation prize is 700*(6/36) = 116.67.
Thus, the average win of the bonus is 1866.67 + 116.67 = 1983.33.